4 Apr 2017: Lab 7: Modeling Friction Forces

Lab 7: Modeling Friction Forces

Joel Cook

Nina Song

Lab performed March 20, 2017

In this lab we performed a series of experiments to model static and kinetic friction forces for different scenarios. 

Introduction: To determine the coefficient of friction for different surfaces and scenarios, we will set up experiments where we know the masses of the objects, angles of the surfaces, or force being applied and can determine the coefficient of friction, for both static and kinetic, from the following equations:

f_s=μ_sN

f_k=μ_kN

f is friction force, μ is coefficient of friction and N is normal. The subscript s denotes static friction and the k denotes kinetic friction.

Static friction exists when the objects are not moving while kinetic friction opposes the motion of two objects moving against one another. 

Procedure: The first experiment in this lab involved calculating the coefficient of static friction by setting up an apparatus in which a block rests on a table top with a string tied to the block, run through a pulley, and tied to a hanging mass, as seen below.

The mass of the block is measured. Mass is added to the hanging portion until the block resting on the table moves. At the point the block moves there is no longer static friction. We recorded the hanging mass at which the block moved, minus 5 grams, as the amount of hanging mass that provided the highest static friction force. We then repeated the process three more times with 200 grams added on top of the block each time. 

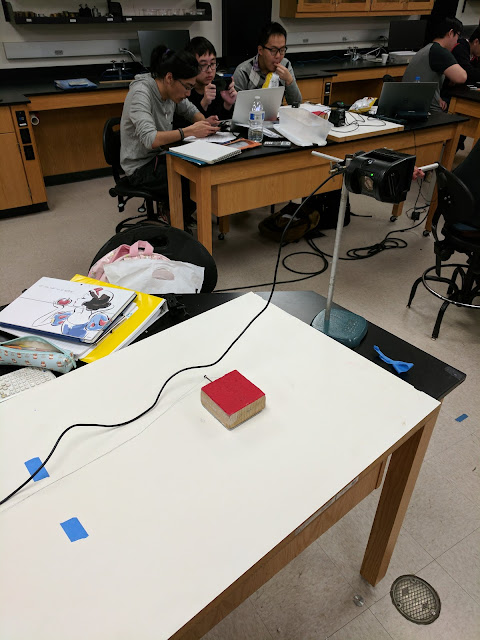
For the second experiment, since kinetic friction is modeled as being proportional to the normal force, we are able to determine the the friction force acting on a block by pulling the block, with a force sensor attached, at a constant speed across a table top. The apparatus used is shown below.

By using a constant speed, the acceleration of the system is zero. The force applied to keep the object moving is equal to the kinetic friction force opposing the motion of the block.

For the third experiment, we placed a block on a surface and slowly raised the surface until the block slid down by it's own weight. We can determine the maximum static friction force by measuring the angle at the point where the block slides. At the point the block just begins to slide, the static friction force is equal to the x-component of the weight of the block.

For the fourth experiment, we attached a motion sensor above an inclined surface to measure the acceleration of the block as it slides down the incline. The apparatus used is shown below.

Since the acceleration of the block is due to the x-component of the weight of the block, the kinetic friction force is equal to:

f_k=m*a-mgsinΘ

f_k=μ_k*N

N=mgcosΘ

For the last experiment, we predicted the acceleration of a two-mass system. The apparatus is shown below. 

We used the coefficient of static friction from experiment four and two known masses. The prediction is shown below.

We then used the motion sensor to measure the acceleration and compared the result to our prediction.

Data:

Experiment 1:

The data for experiment one is shown in the following table, where the hanging mass is the mass at which the block slid, minus 5 grams:

Mass of Block (g)	Hanging Mass (g)
182	105
382	205
582	380
782	500

We plotted static friction force, in newtons, versus normal force, in newtons. The data was plotted in Logger Pro and the slope of the line through the data points is the coefficient of static friction. The calculation below shows how we calculated static friction and normal force. 

f_s-T=m*a=0

T=Mg

Therefore, f_s=Mg

And f_s=μ_s*N where N=mg

Static Friction Force (N)	Normal Force (N)
1.029	1.7836
2.009	3.7436
3.724	5.7036
4.9	2.6636

The graph is shown below. The coefficient of static friction was 0.6283.

Experiment two:
For experiment two, the data gathered is shown below:

Pulling Force (N)	Mass of Block (g)
0.5077	182
1.093	382
1.652	582
2.230	782
2.743	982

The pulling force was determined by analyzing the results from logger pro for pulling the block along the table for the average force over the time period. An example of this data is shown below.

The above photo shows the data for pulling force versus time for all of the trials. To determine the coefficient of kinetic friction, the data was graphed as before for static friction. The data was graphed as kinetic friction force versus normal force with the slope of the line through these points being the coefficient of kinetic friction.

f_k=μ_k*N

N=mg

The coefficient was 0.2880.

Experiment 3:

Three trials were performed. The block slid at 26.8°, 30.2°, and 32.6° for an average value of 29.9°. The coefficient of static friction was calculated as shown:

Experiment 4:

We measured the acceleration of the block on the incline with a motion sensor and Logger Pro. The graph below shows the acceleration that was measured as 0.7433 m/s^2. The incline of the surface was measured using a smartphone application at 22°.

By using this value for acceleration we were able to calculate the coefficient of kinetic friction for the trial, as shown below. The calculated coefficient was 0.322. 

Experiment 5:

For the last experiment, we used the value calculated in experiment 5 to predict the acceleration of a two-mass system. The mass of the block was 0.182 kg and the hanging mass was 0.2 kg. As shown, we predicted the acceleration would be 3.63 m/s^2.

We measured the acceleration of the block using a motion sensor as before at 3.771 m/s^2, as seen in the graph.

Since the measured value was within 5% of our predicted value, our prediction was accurate.

Conclusion: In this lab, we set out to model static and kinetic friction forces. Based on the accuracy of the predictions we made and the results of our various experiments, our lab was successful. There were many sources of uncertainty in our experiments. Modeling friction assumes the coefficient of friction is consistent on the surface being tested and this is not possible in our conditions. Our surfaces were not perfectly flat, clean, uniform or smooth and this will affect the friction force. For our purposes, the conditions were acceptable. In addition, it is always possible that trials are performed inconsistently which would affect the accuracy of results.